Dilution

Overview

Dilution is achieved by adding a specified volume of a liquid material of known concentration to an appropriate volume of a solvent liquid.

1 in 10 dilution

The video below explains the concept of 1 in 10 dilution.

This video contains sound.

Let's suppose that we have Copper Sulphate (CuSO₄) solution with concentration C₁. If we take 1 part of this solution and dilute it by adding it to 9 parts of a diluent e.g. distilled water, the resulting solution will make 10 parts altogether with a new concentration of C₂. Such a dilution is called 1 in 10 dilution, where 1 part of an existing solution is diluted with 9 parts of a diluent e.g. distilled water to give 10 parts. The word part refers to the same unit. So this can be:
1 millilitre + 9 millilitres = 10 millilitres
Or 1 litre + 9 litres = 10 litres and so on.

The concentration of Copper Sulphate in the new solution has been reduced by a factor of 10, hence the dilution factor is 10. If the original concentration C₁ was 0.5 Molar, concentration of Copper Sulphate in the new solution will be 0.5 Molar divided by 10 which is equal to 0.05 Molar. Similarly if the original concentration was 0.05 Molar, then the concentration after 1:10 dilution will be 0.05 divided by 10 which is equal to 0.005 Molar.

For a 1:10 dilution, the amount of original solution and the diluent can be any amount as long as the original solution and diluted solution are in a proportion of 10.
So this can be 10 mL of Copper Sulphate solution diluted with 90 mL of distilled water giving 100 mL.
Or it can be 2.5 mL of Copper Sulphate solution diluted with 22.5 mL of distilled water giving 25 mL.
Or it can be 75 mL of Copper Sulphate solution diluted with 675 mL of distilled water giving 750 mL.

In case of 1 in 5 dilution, the amount of original solution and diluted solution should be in proportion of 5.
This can be 1 mL of Copper Sulpahte solution diluted with 4 mL of distilled water giving 5 mL.
Or 10 mL of Copper Sulpahte solution diluted with 40 mL of distilled water giving 50 mL
Or 2.5 mL of Sodium Chloride solution diluted with 10 mL of distilled water giving 12.5 mL and so on.

We know that:

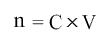
where n is the amount of solute in moles, C is Concentration and V is the volume of solution in litres.

In the above example, as both the solutions have the same quantity, n, of CuSO₄ therefore the product of C and V in the original solution remains unchanged in the diluted solution. Therefore:

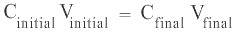
C initial times V initial is equal to C final times V final

The above equation can be re-written as:

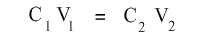
where C₁ and V₁ are initial (i.e. before dilution) concentration and volume respectively and C₂ and V₂ are final (i.e. after dilution) concentration and volume respectively.

Note that in the above formula it is not important which units are used for C and V, but it is important that the same units are used for C₁, V₁ and for C₂, V₂. You can use the above formula to handle more complicated problems. The example in the next section demonstrates the use of this formula.